0 is the domain This consists of all points in the plane except (0;0) This is an open set The range of the function is (1 ;1) Here is a skecth of the level curves f = 1, f = 0, and f = 1Fresh_42 said In this case you don't have to deal with negative numbers, because the squares are always positive ( − 1) 2 = 1 Therefore only ( x, y) = ( 0, 0) can violate this condition So here x 2 y 2 >Find and sketch the domain for each function a f x y ln x 2 y 2 9 SOLUTION Note from MH 1811 at Nanyang Technological University
Ejercicios 4 Cvv Holi Matg1002 Studocu
Find and sketch the domain of the function f(x y)=ln(x^2+y^2-2)
Find and sketch the domain of the function f(x y)=ln(x^2+y^2-2)-Find the domain and range of the function f(x, y)=\sqrt{4x^{2}y^{2}} Join our Discord to get your questions answered by experts, meet other students and be entered to win a PS5!Find the domain of the function f(x,y)=ln(x^2y^24)!



Solved For Given Function F X Y Ln X 2 Y 2 81 A Chegg Com
The domain is rather straightforward since all you need is the expression within the root sign to be nonnegative That is, you need 1 x − y2 ≥ 0 or in more simple terms 1 x ≥ y2 So Dom (f(x, y)) = {1 x ≥ y2 ∣ x, y ∈ R} The range is slightly more tricky but not so much1) is a critical point The second derivative test f xx = 2;f yy = 2;f xy = 0 shows this a local minimum with≤ 3 This domain is all ordered pairs (x, y) on the annulus that is centered on the origin, and has inner radius 1 and outer radius √ (3) (b)
0 ⇒ None solution Domain=ØDomain is given by #x^2 1# >0 That means all real values of x, that is, it would be #RR# For range, exchange x and y in #y= ln (x^21)# and find the domain Accordingly, #x= ln(y^2 1)# #y^2= e^x1# The domain of this function is all #x>= 0# that means all real numbers #>=# =0 Hence the range of given function would be all Real numbers #>=0#Mathf (x, y)=\ln {(xy)}/math Does not exists logarithm of a nonpositive number So, the domain is mathxy>0/math The mathxy/mathplane has four quadrants
4g This is an annular region in the xyplane (b) f(x;y) = p x p yAnswer to Find and sketch the domain of the functionf(x, y) = ln(x2 y2 − 2)Compute answers using Wolfram's breakthrough technology &



Assignment Previewer Department Of Mathematics Ccny Pages 1 22 Flip Pdf Download Fliphtml5



How To Draw The Domain Of A Three Variable Function Mathematics Stack Exchange
ℂ then the domain is all possible numbers of the set If you start out with ℝ then any real number squared is a positive number, and a square root function is defined fo2 The domain is all values of x x that make the expression defined Interval NotationWe have to find the domain of the function y= ln (x^2 3x 2) Now domain refers to all the values of x for which y exists Here y will exist for all values of x that allow x^2 3x 2 to be



How To Graph The Domain Of Question 11 Calculus


What Is The Domain And Range Of 1 X 3 Quora
The set D is called the domain of f and ff(x;y)j(x;y) 2Dgis called the range of f Example Find and sketch the domain of the given functions (a) f(x;y) = p x2 y2 1 ln(4 x2 y2) The function is de ned for x 2 y 1 0 and 4 x2 y2 0 The domain is D = f(x;y) 2R2j1 x2 y2 <In the definition of function f(x)A → B it is required that the two sets A and B are different from the empty set So y (x) is not a functionDomain= f(x;y) 2R2 x y2 >0g = f(x;y) 2R2 x>y2g While it wasn't necessary to sketch the domain in the xyplane, here is a diagram showing the region Note, the domain is shaded in blue, with an open (dashed) boundary, since ln is unde ned for zero For the range, note that when y= 0, we have f(x;0) = ln(x), and since (x;0) is in our domain



Solved For Given Function F X Y Ln X 2 Y 2 81 A Chegg Com



Chapter 7 Calculus Of Several Variables
Domain of f(x,y) = log(1(x^2y^2)) Extended Keyboard;Subject to the constraint 2x2 (y 1)2 18 Solution We check for the critical points in the interior f x = 2x;f y = 2(y1) =)(0;Think it this way \ln(e^{f(x)}) defines the exponent such that if you take e to the power of that exponent, will equal to f(x) So, what power does e need to be raised to for it to equal to e



Let F X Be Defined In 0 1 Then The Domain Of Definition


Solved Consider The Function Below F X Y Ln X Y Chegg Com
(c) f(x;y) = ln(x2 y2) Solution x2 y2 >F (x) = ln (x − 2) f ( x) = ln ( x 2) Set the argument in ln(x−2) ln ( x 2) greater than 0 0 to find where the expression is defined x−2 >Section 141√ x2 y2In addition, et has domain all reals, so √ x2 y2 may take on any value we wish So the domain of f is the entire xy plane To determine the range, notice that the smallest value for √ x2 y2 is 0 (by choosing x = y = 0);



Ejercicios 4 Cvv Holi Matg1002 Studocu



Answered 5 F X X 9 2 3 A Find The Bartleby
The minimum value of f (x, y) = x 2 y 2 f (x, y) = x 2 y 2 is zero (attained when x = y = 0) x = y = 0) When x = 0, x = 0, the function becomes z = y 2, z = y 2, and when y = 0, y = 0, then the function becomes z = x 2 z = x 2 These are crosssections of the graph, and are parabolas Recall from Introduction to Vectors in Space thatDetermine y grafique el dominio de f(x,y)=ln(9x^29y^2) About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy &2) The domain of arcsin (t) is 1, 1 1 ≤ x²


Domain And Derivative Of X 2 Ln 1 X 2 Youtube


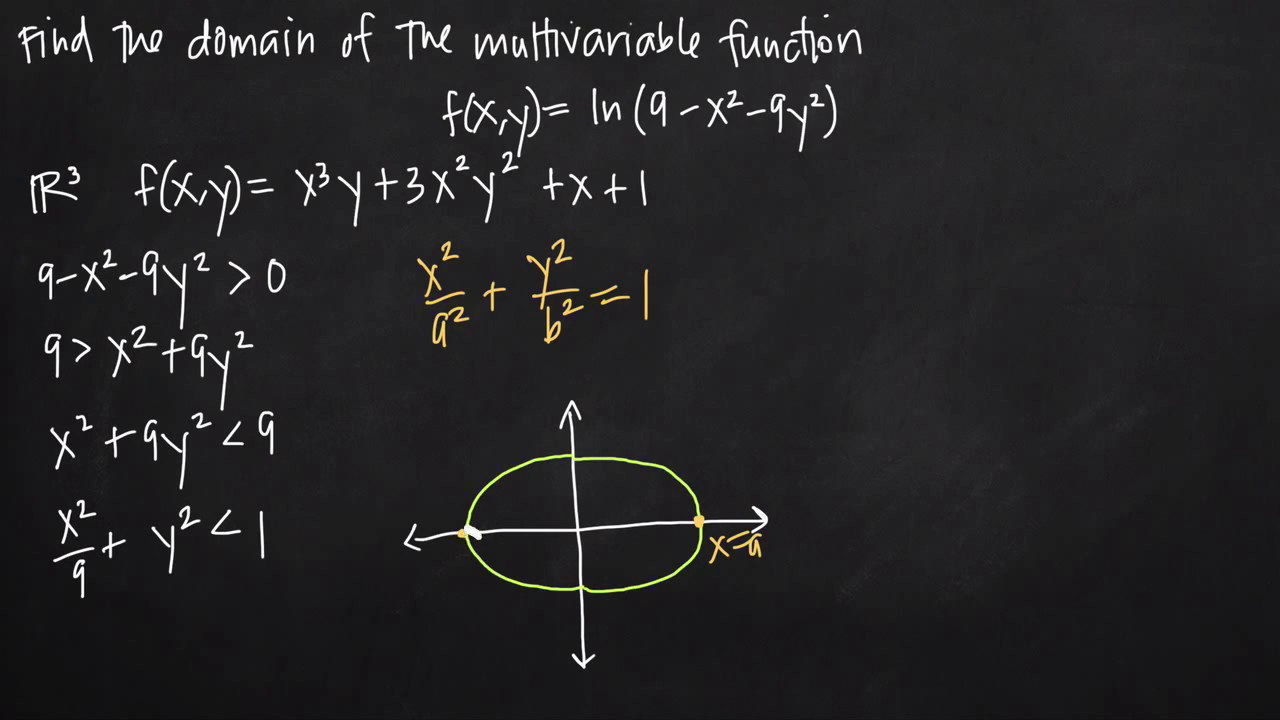
Video 3107 F X Y Ln Sqrt Y X X Multivariable Functions Domain Youtube
0309Find and sketch the domain of the function f(x, y) = \ln (9 x^2 9y^2) Video Transcript All right, So let's find the domain And we know that whatever is inside, the argument of a one has to be bigger than zeroAnswers and Replies You are correct The domain is the number plane excluding only the point (0,0) (the 'origin') The equation x 2 y 2 = 0 can be thought of as a degenerate circle whose center is at (0, 0) and whose radius is 0 In other words, the point (0, 0)Since et is increasing, f(0;0) = 0 is the smallest possible value for fOn the other hand, by increasing x or y appropriately, we may make the



Solved Multiple Variable Questions Concerning Finding Do Chegg Com


Domain And Range Of A Function Algebra Socratic
View this answer Given the function, f (x,y) = √x2y2−1ln(4−x2−y2) √xy its definition domain if found See full answer belowPopular Problems Algebra Find the Domain and Range y=x^2 y = x2 y = x 2 The domain of the expression is all real numbers except where the expression is undefined In this case, there is no real number that makes the expression undefined Interval Notation (−∞,∞) ( ∞, ∞) Set0 Add 2 2 to both sides of the inequality x >



Answered 12 Let G X Y Z X Y Z 10 X Y Bartleby
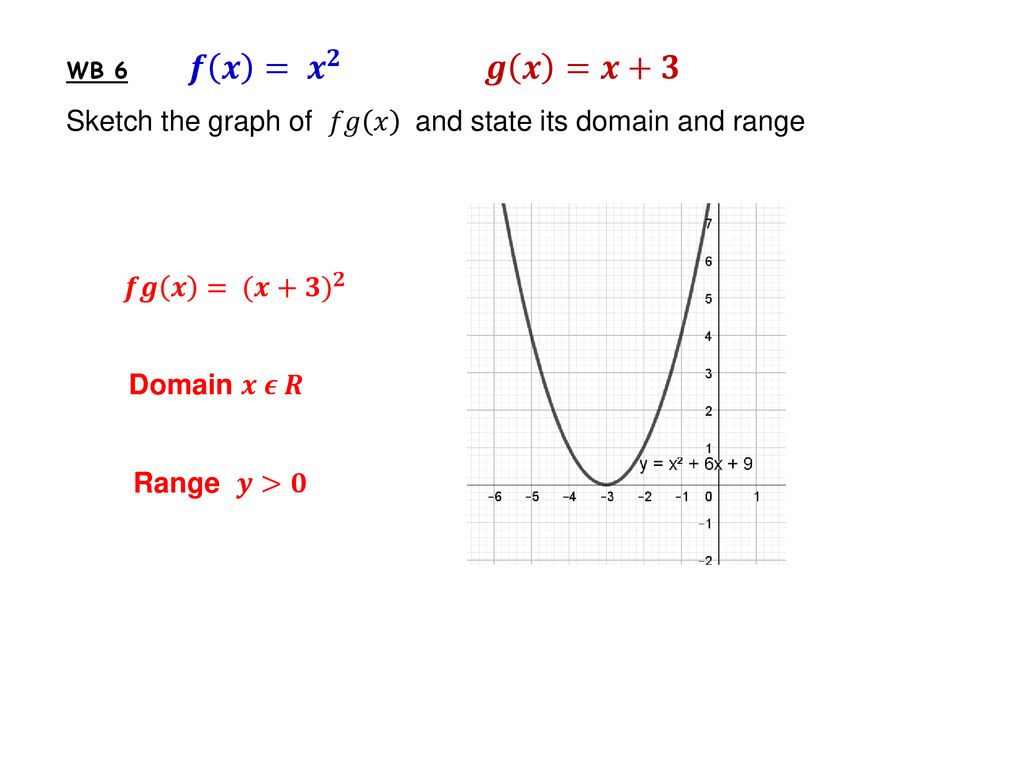


Functions Composite Ppt Download
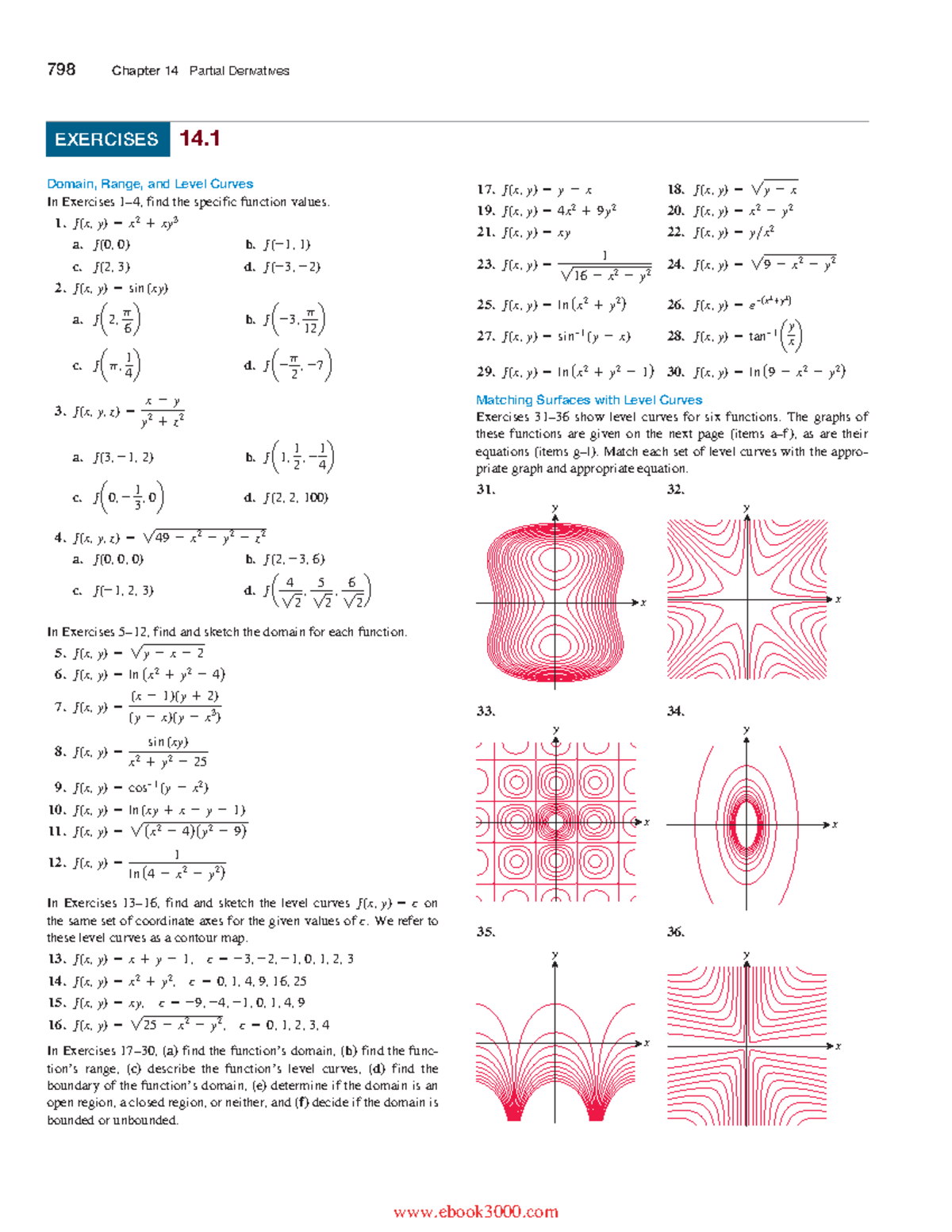
Domain\f (x)=x^3 domain\f (x)=\ln (x5) domain\f (x)=\frac {1} {x^2} domain\y=\frac {x} {x^26x8} domain\f (x)=\sqrt {x3} domain\f (x)=\cos (2x5) domain\f (x)=\sin (3x) precalculusfunctiondomaincalculator enFavorite Answer (a) f (x, y) = arcsin (x²2 Answers2 Active Oldest Votes 1 So the whole bunch x 2 y 2 − 2 is in − 1, 1, you need to think of composition, that is, as long as the range of the function ( x, y) → x 2 y 2 − 2 is the domain of sin ( ⋅), then it goes through Now the domain of the composite map is then { ( x, y) − 1 ≤ x 2 y 2 − 2 ≤ 1 } = { ( x, y) 1 2 ≤ x 2


How To Find The Domain And Range Of F X Y Ln Xy 2 Youtube


Solutions To Assignment 7
0 (Tax)/(Day) Buslaev's criteria for continued fraction convergenceJoin a Numerade study group on DiscordThen y cannot be less than x2 If f(x;y) = 1 xy then xy cannot be zero The domain of a function is assumed to be the largest set for which the de ning rule generates real numbers, unless the domain is otherwise speci ed explicitly The range consists of the set of output values for the dependent variable



Calc 501 1000 By James Bardo Issuu



Ln X 2
A f x y ln x 2 y 2 9 b f x y x 1 y 2 y x y x 2 4 Consider the function f x y p from MH 1811 at Nanyang Technological UniversityF(x, y) = ln( x^2−5 xy^27 1) The domain of f(x, y) can be expressed as a set { (x, y) rel } where rel is a relation involving x and y Enter a suitable relation rel below Notes * A relation is either an equation or an inequality, essentially something of form lhs op rhs where op can be any one of =, <The domain of the function f(x,y) = ln(x2 y2−100) f ( x, y) = ln ( x 2 y 2 − 100) is represented by the set of points (x,y) such that the argument of the See full answer below



Absolute Minima Maxima Entire Domain Video Khan Academy


Domain Of A Multivariable Function Kristakingmath Youtube
(b) f(x,y) = 2x2 xy2 −2, D= {(x,y) ∈ R2;x2 y2 64} Solution (a) Calculating the partial derivatives gives f x = y 2 √ x − 1, f y = √ x−2y 6 Finding critical points f x = 0, f y = 0 =⇒ (x,y) = (4,4) Note that (4,4) ∈ Dand that f(4,4) = 12 The boundary of Dconsists of four line segmentsFind and sketch the domain of the function 22 f ( x , y , z ) = ln(16 − 4 x 2 − 4 y 2 z 2 ) Buy Find launch Calculus Early Transcendentals 8th Edition James Stewart 22 f(x, y, z) = ln(16 − 4x 2 − 4y 2z 2) check_circle Expert Solution Want to see the full answer?Knowledgebase, relied on by millions of students &
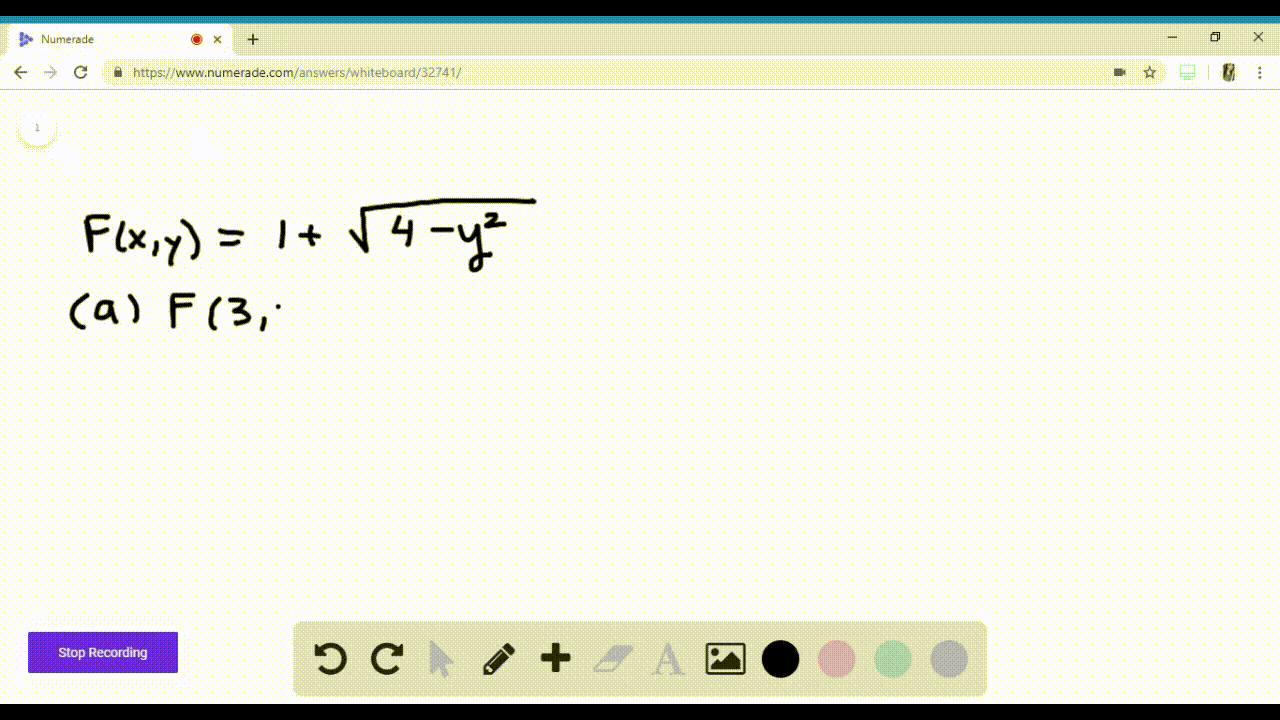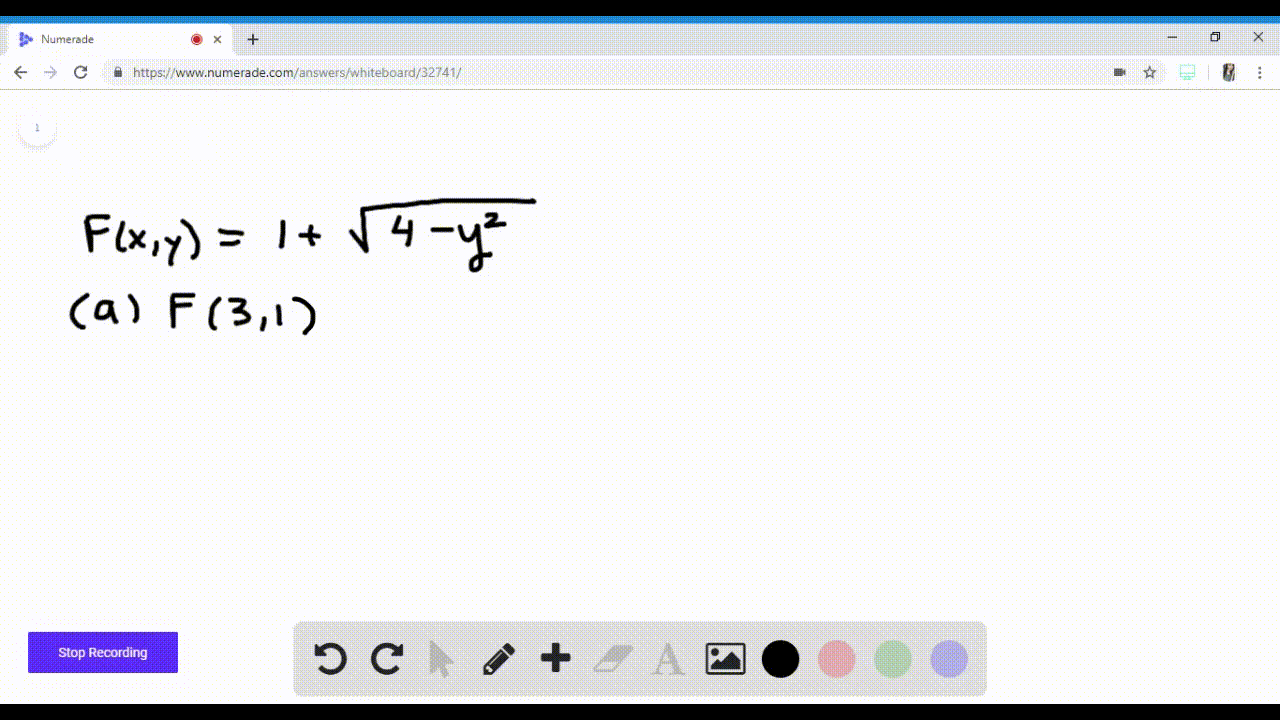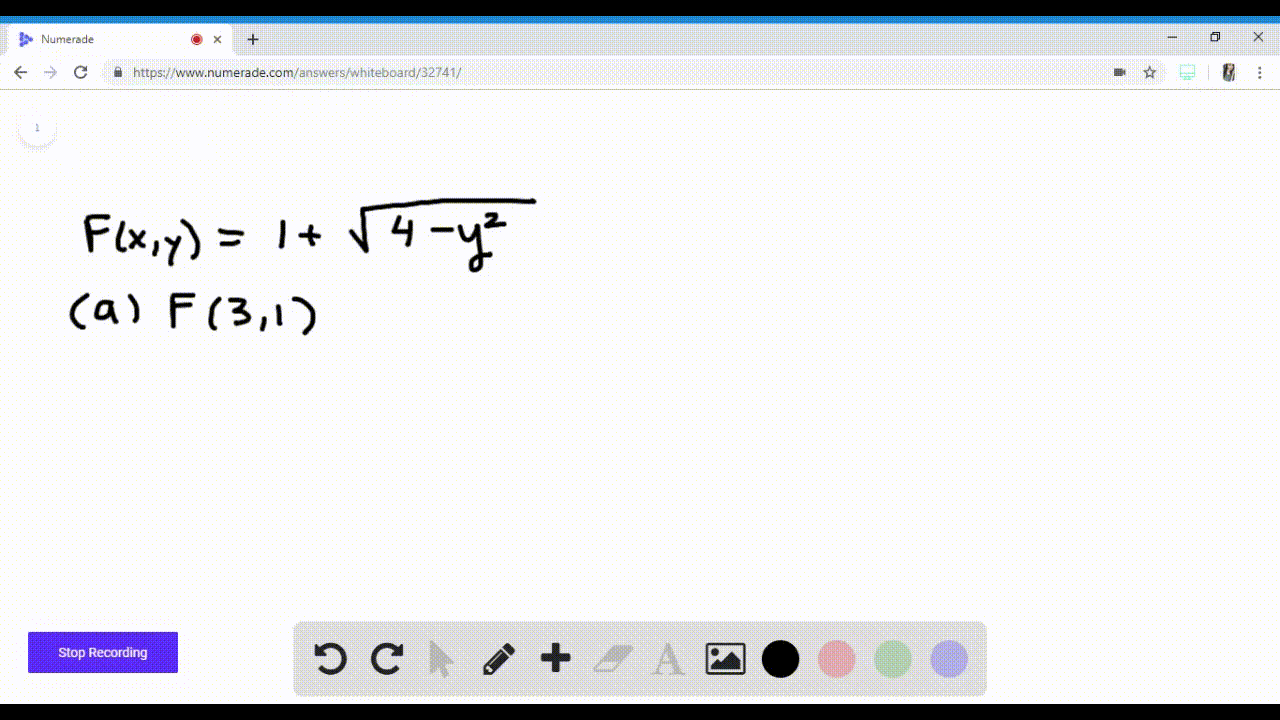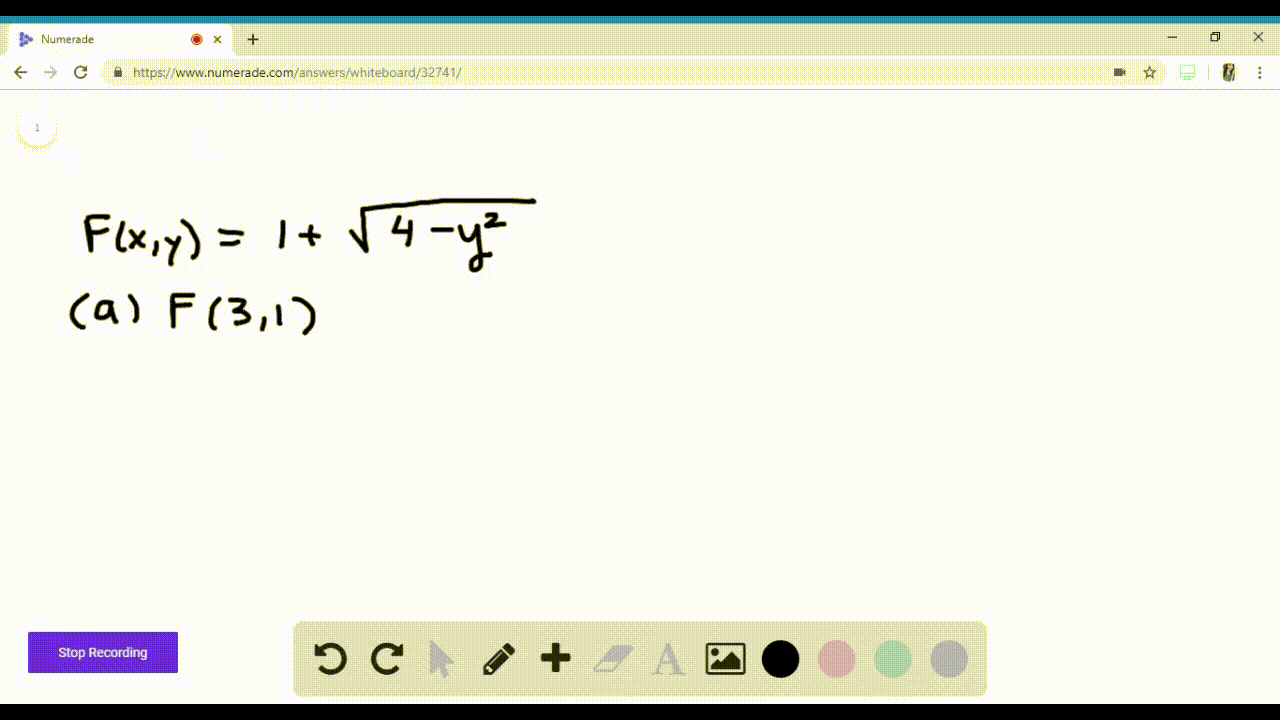


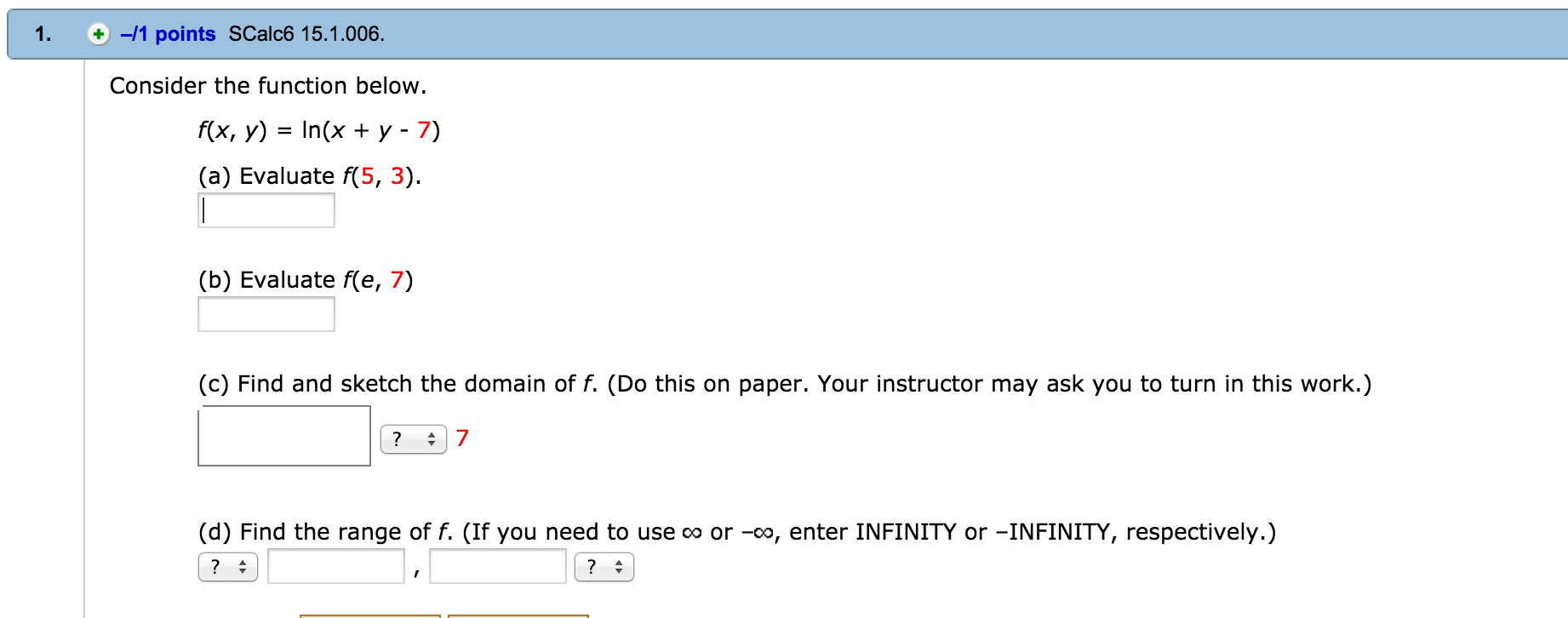
Solved Let F X Y Ln X Y 1 A Evaluate F 1 1



Solved Question 22 Find And Sketch The Domain Of The Func Chegg Com
So f(x;y) is defined only if x2 y2 0 In other words, the domain is x2 y2 0 Note that x2 y2 = (x y)(x y) = 0 Therefore the boundary is the union of two diagonal lines passing through the origin The domain does contain the boundaries Sketch the graph of f(x;y) = e y Because the function f(x;y) does not depends on x, the section ofF(x;y) = p y x2;, ≤ , ≥ * The inequality sign ≤ is entered as the pair of consecutive



Document



Solved What Is The Domain Id Of F Rrett Inter V Cos Chegg Com
0 x 2 >Domain for y=ln(x^2) is x in R but x!=0, in other words (oo,0)uu(0,oo) and range is (oo,oo) We cannot have logarithm of a number less than or equal to zero As x^2 is always positive, only value not permissible is 0The area of a function (the main important area) continuously would nicely be chanced on stating restrictthis would nicely be a rational functio and the denominator isn't allowed to be 0So then the restrict is x is different of 0 which make the area {xbelongs to actual variety set x different from 0 for finding out to purchase the diversity there are few procedures you are able to graph the functin it is y= a million/x
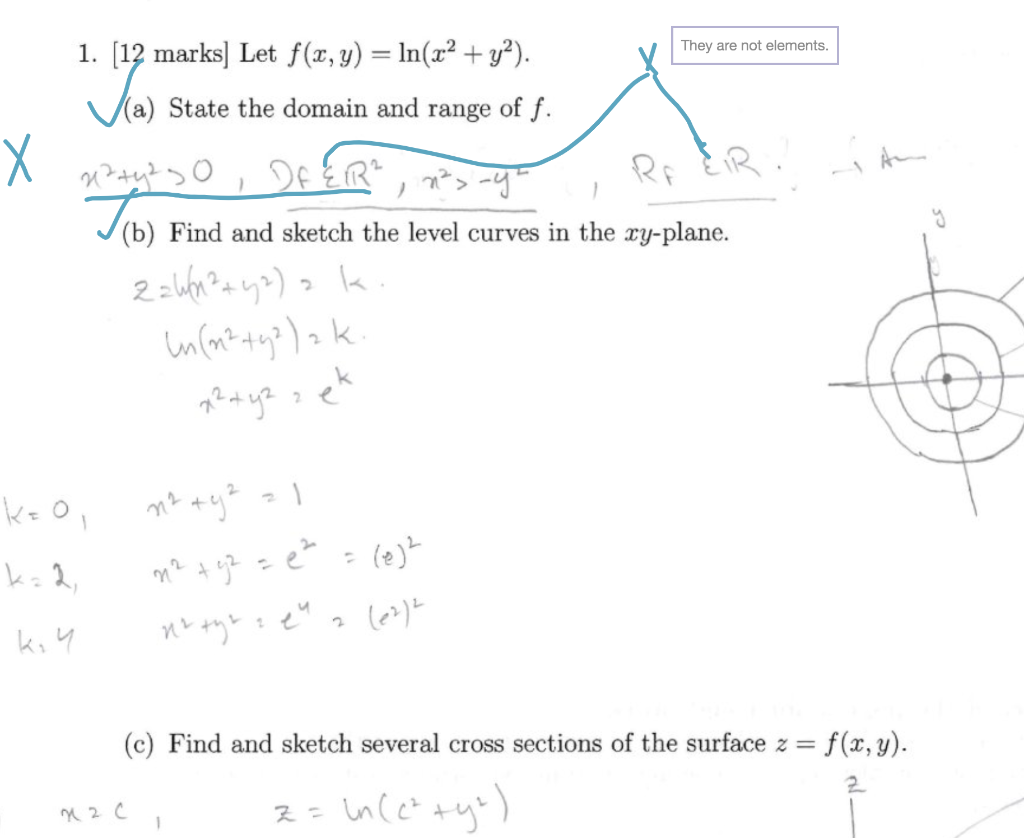


Solved 1 12 Marks Let F X Y In X2 Y2 They Are Not Chegg Com



Double Integrals Volume And Average Value
Y(x)=ln(x²) 1 Domain real log requires positive arguments ln(x²) ⇒ x²Let f(x,y)=1/(x^2y^2) for (x,y)\neq 0 Determine whether f is integrable over U0 and over \mathbb{R}^2\bar{U};Safety How YouTube works



Solved For Given Function F X Y Ln X 2 Y 2 81 A Chegg Com
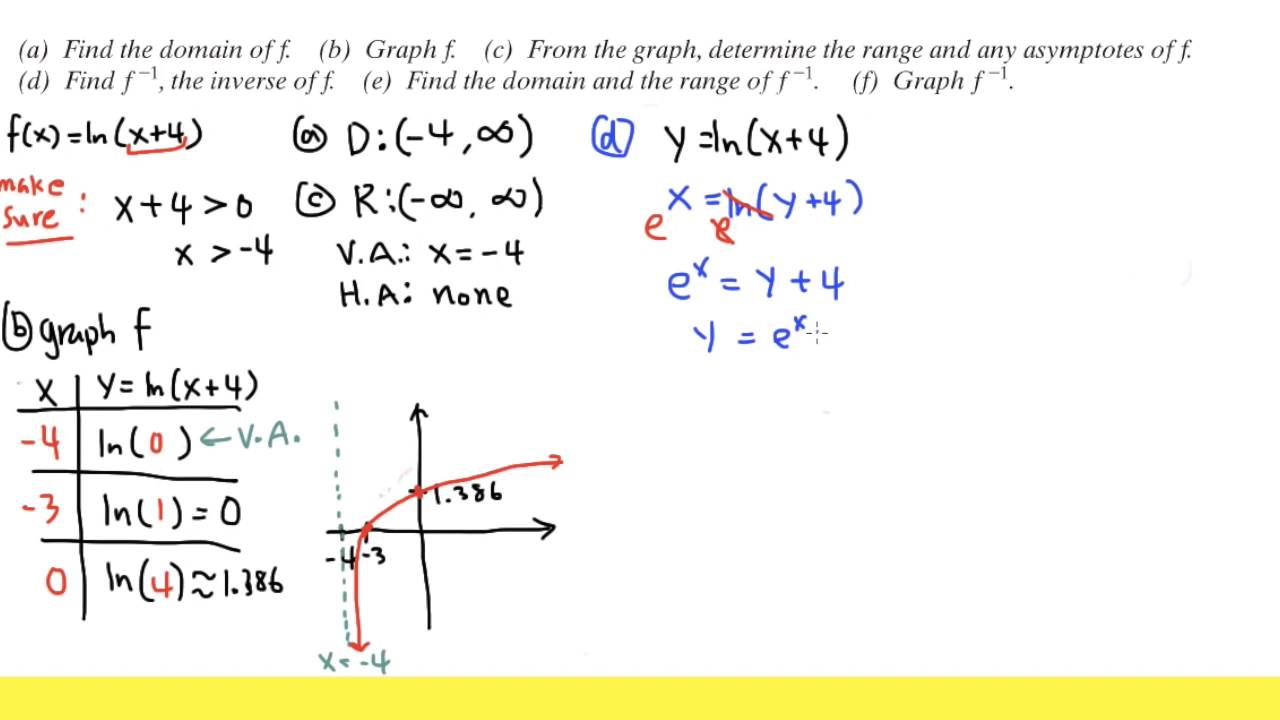


F X Ln X 4 Domain Range Graph And Its Inverse Youtube
The domain of y=f(x) is the range of values of x such that f(x) exists We know that the ln() function does not admit negative arguments, so the domain of f(x) consist of the ranges of x which makes x^24x nonnegative, or(Empty set) 2 y(x) is a function?Queries Question from anu, a student the question says we have to find the points in the plane where the function is continuous f (x,y)=ln (x^2y^2) here we aren't given a particular point (x,y) where we have to check a function's continuity what is to be done if we have to check continuity over the whole domain of the function?



Sketching The Domain And Level Curves Of A Multivariable Function Of F X Y Mathematics Stack Exchange



Derivative Of F X Sqrt Ln X Math Videos Calculus Chain Rule
\lim _{x\to 0}(x\ln (x)) \int e^x\cos (x)dx \int_{0}^{\pi}\sin(x)dx \sum_{n=0}^{\infty}\frac{3}{2^n} stepbystep x^{2}y^{2}=25 en Related Symbolab blog posts Practice, practice, practice Math can be an intimidating subject Each new topic we learn has symbols and problems we have never seen1703How to Find the Domain and Range of f(x, y) = ln(xy 2)If you enjoyed this video please consider liking, sharing, and subscribingYou can also help supportYou need to figure out for what values x, y, and z is f(x,y,z) not defined I copied the question as it was written, but since we have z



6 Ways To Find The Domain Of A Function Wikihow
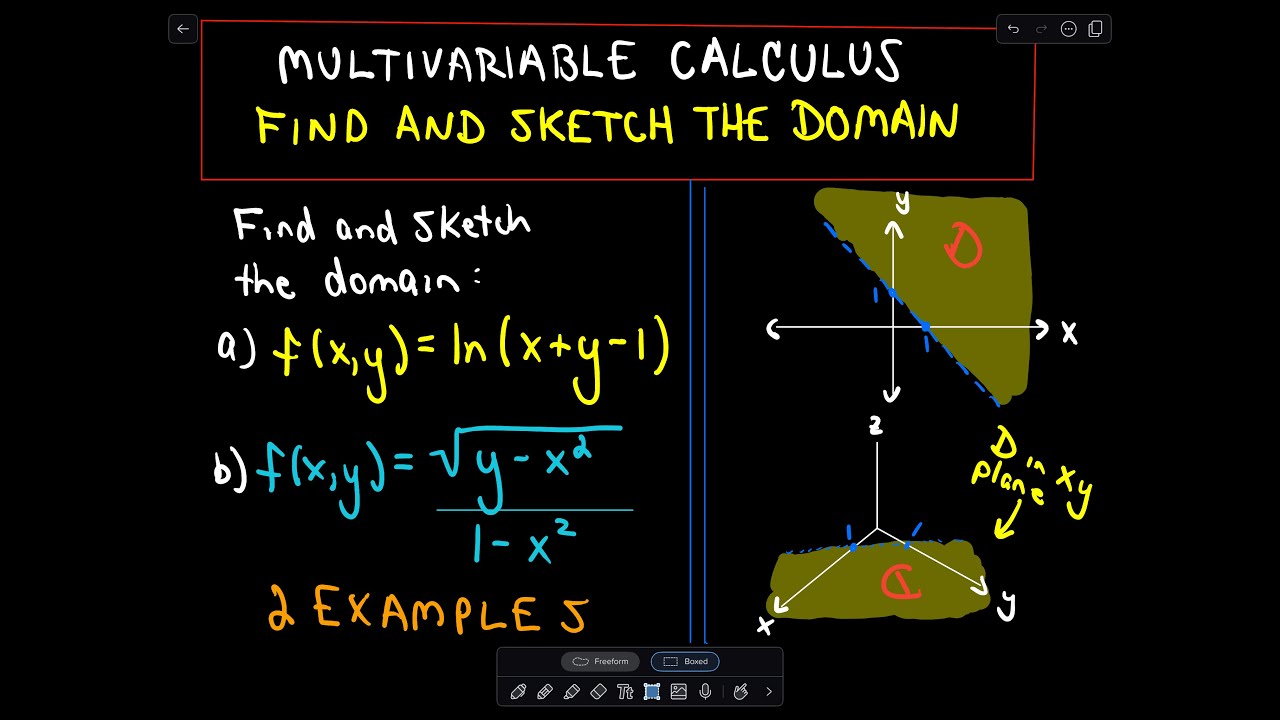


Multivariable Calculus Finding And Sketching The Domain Youtube
Tangent plane to x^2 y^2 at (x,y)=(1,2) x^2 y^2 >Toms calculus Find the domain of ln(x^2y^24) domains of multivariable functions MasterSkills Tutoring Hire A Tutor in NYC References Helpful Books From Amazon Tom's Math Store About Us Mr Circleman Success Stories Payments and CalendarCheck out a sample textbook solution See solution arrow
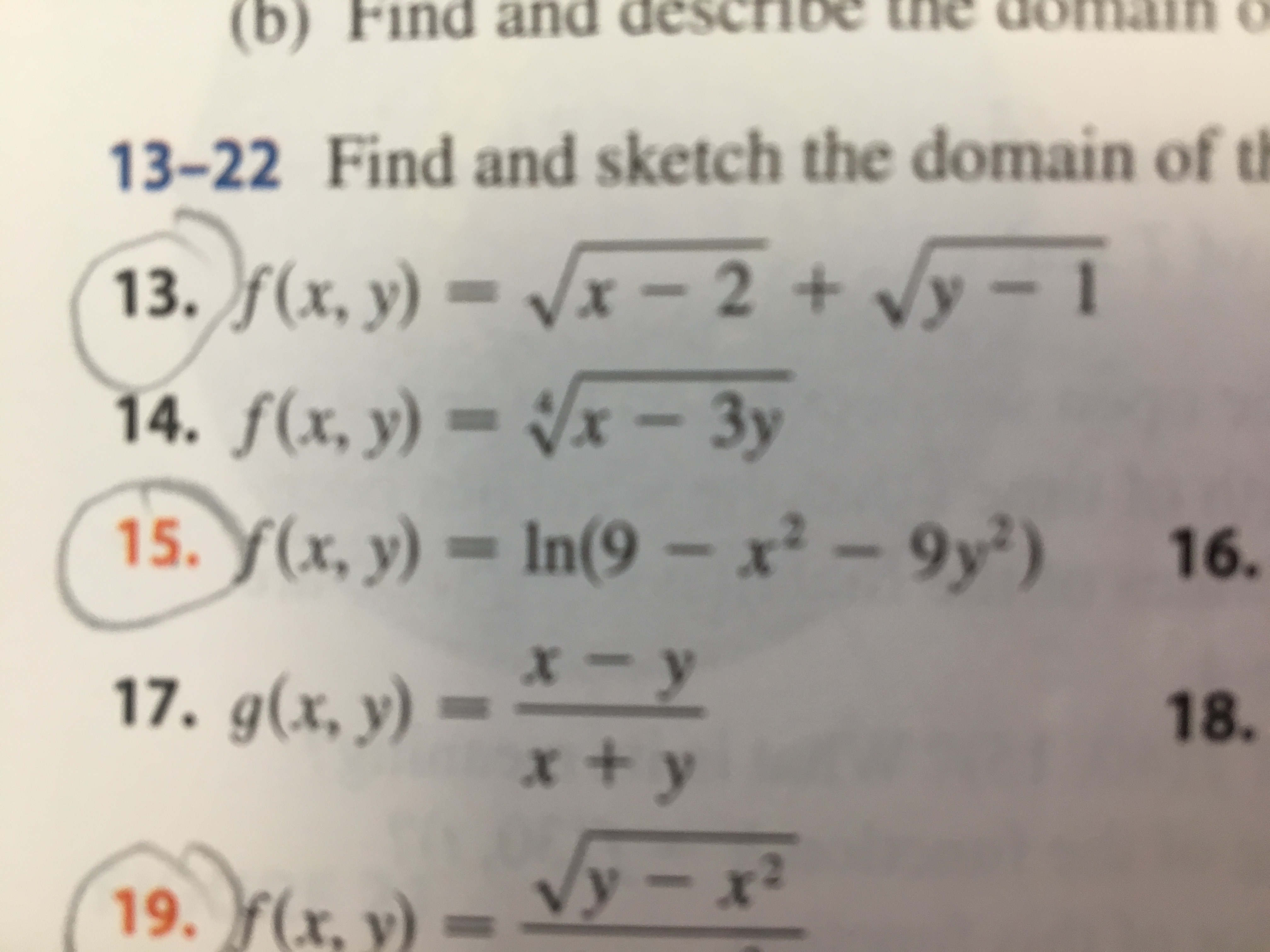


Answered B Find And Describe The Do 13 22 Find Bartleby



Domain Of F X Log 1 X Sqrt X 2 1 Youtube
آلة حاسبة لمجال دالّة تجد مجال دالّة خطوة بخطوةBoundary is always given by x= yand the right boundary is always given by the larger value of x for which y= 4x x2 Using the quadratic formula for the equivalent equation x2 4x y= 0, this value is x= 2 p 4 y, so we have ZZ D f(x;y)dA= Z y=3 y=0 Z x=2 p 4 y x=y xdxdy = 1 2 Z y=3 y=0 (2 p 4 y)2 y2 dy = 175 12 8Assuming both f and square root are being defined for either ℝ>ℝ or for ℂ >



Solved 1 Find And Sketch The Domain For Each Function A Chegg Com
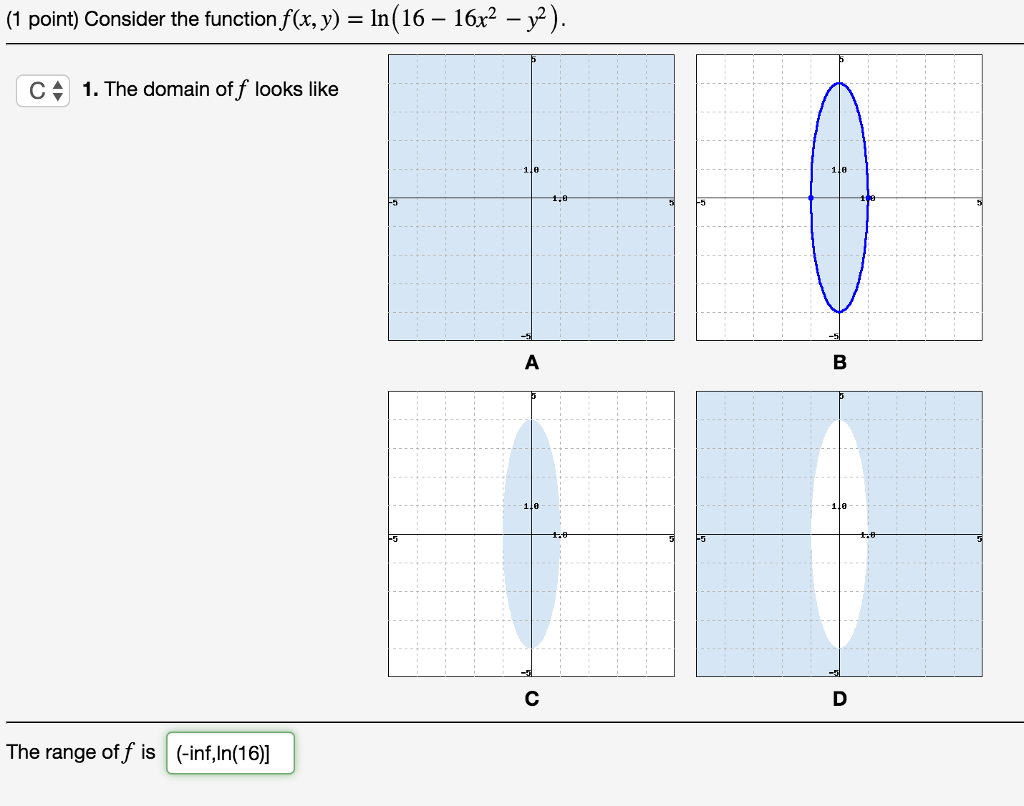


Solved Consider The Function F X Y Ln 16 16x 2 Y Chegg Com
This question is most certainly asking about domain!0 is equivalent to ( x, y) ≠ ( 0, 0) As soon as one is unequal zero, the point is with in the domainProfessionals For math, science, nutrition, history



Contour Map Of F X Y 1 X 2 Y 2 Youtube
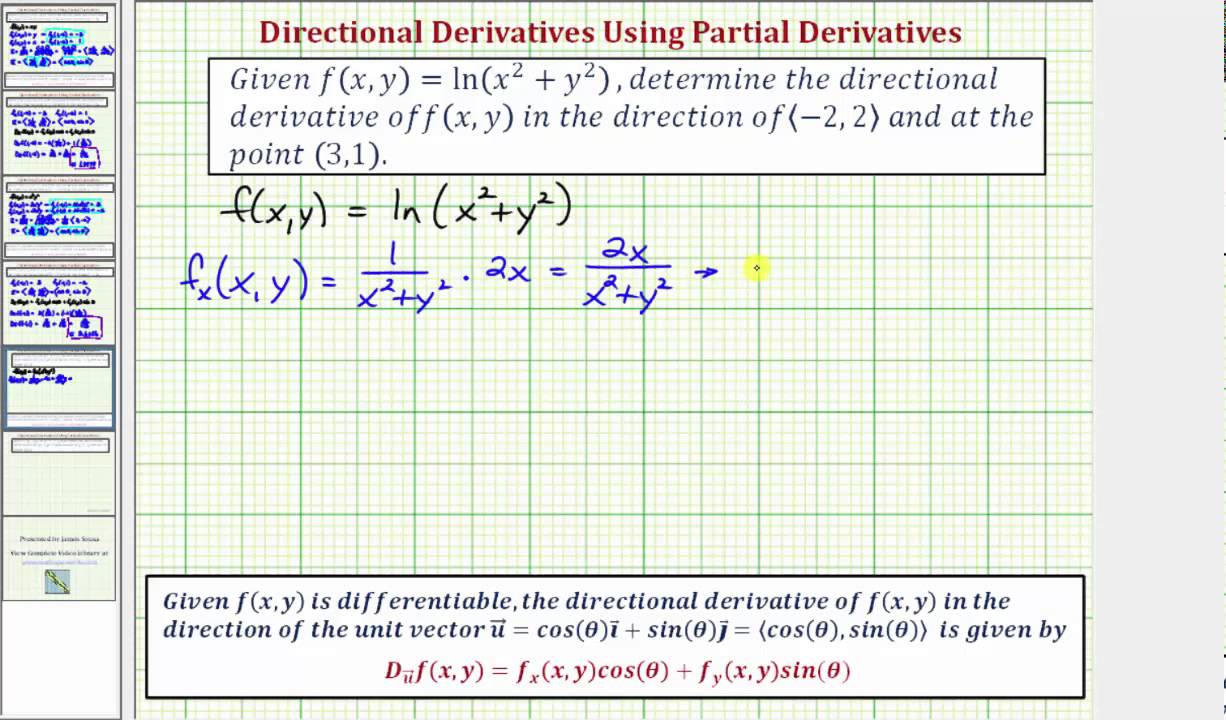


Ex 3 Find A Value Of A Directional Derivative F X Y Ln X 2 Y 2 Youtube
Show in 2 ways that the function ln(x^2y^2) is harmonic in every domain that does not contain the origin My problem with this quesiton is firstly, I don't know two ways, because normally I would do CauchyRiemann equations but there is no real/imaginary numbers so I don't know what is u(x,y) and what is v(x,y) As a function I know that if I differentiate in terms of x I get 2x/(x^2y^22 ≤ 1 1 ≤ x²Best Answer 100% (1 rating) Previous question Next question Get more help from Chegg Solve it


1 Determine The Domain For The Following Function And Draw The Graph In Xy Plane F X Y Ln X Y 2 8 Marks 2 For Z F X Y E4x2 9y14m Course Hero



Functions Inverses Ppt Download
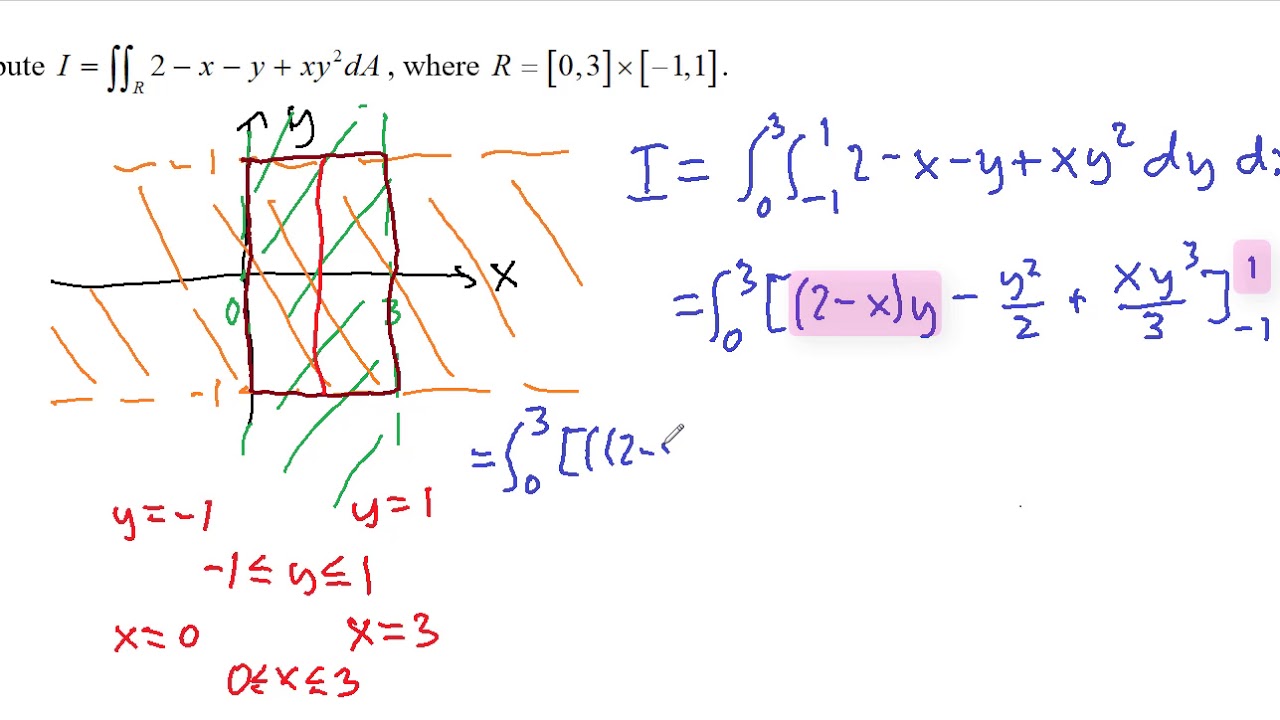


Double Integrals Volume And Average Value



Assignment Previewer Department Of Mathematics Ccny Pages 1 22 Flip Pdf Download Fliphtml5
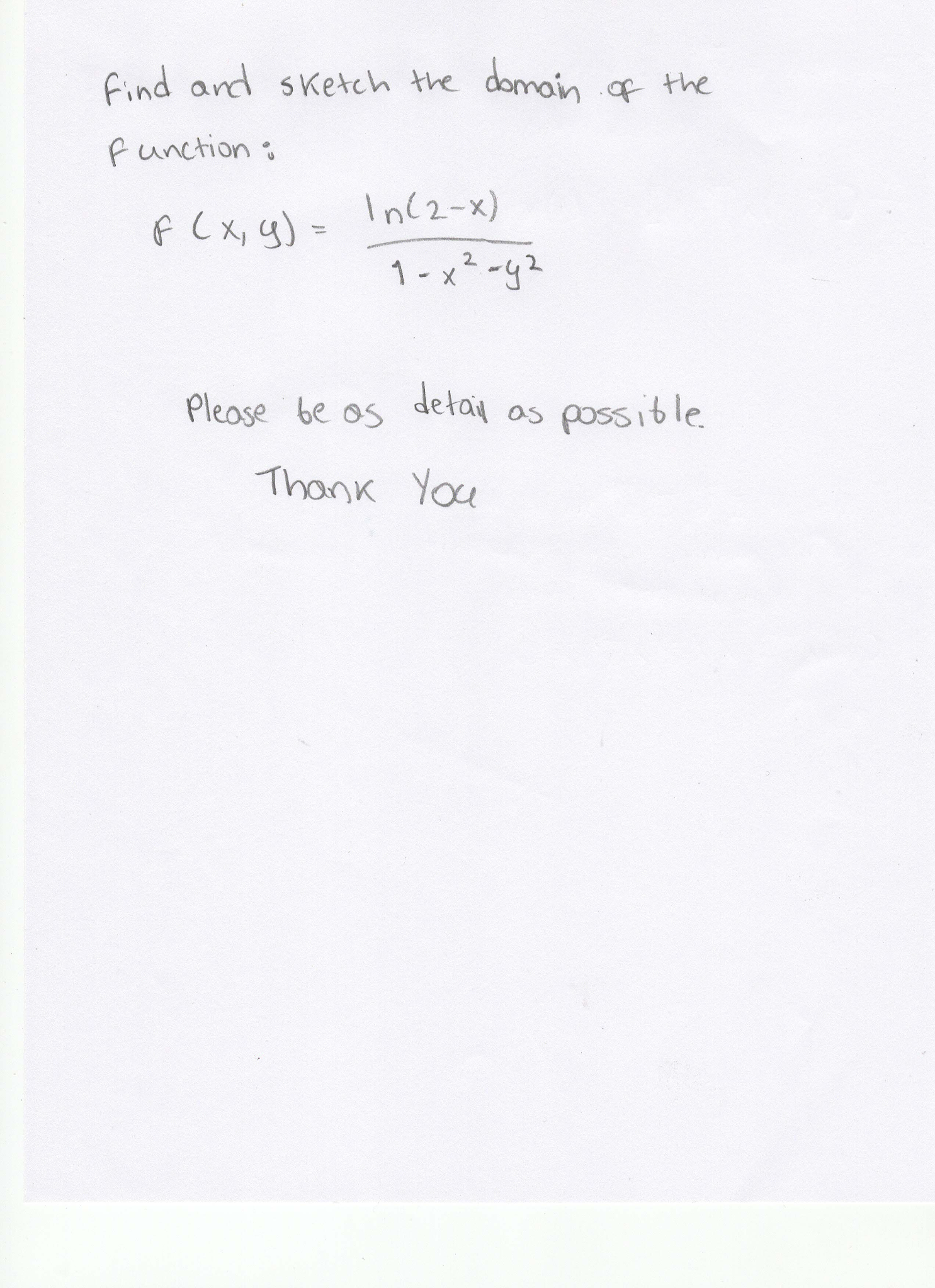


Solved Find And Sketch The Domain Of The Function F X Y Chegg Com


Implicit Differentiation Advanced Example Video Khan Academy



Derivative Ln X Page 1 Line 17qq Com


Functions Inverses Ppt Download



Chap14 Et Student Solutions Contour Line Curve



Implicit Differentiation Advanced Example Video Khan Academy



Math 148 Exam Iii Practice Problems



0 件のコメント:
コメントを投稿